Quantum Field Theory

Research Strand:

Fields and Strings (Theoretical Physics)

Quantum Field Theory (QFT) is an overarching framework in theoretical physics. It describes quantum systems with degrees of freedom at every point in space, that interact with each other locally. Its applications range from statistical and condensed matter physics, to particle physics, cosmology and quantum gravity.

QFT is extremely successful in providing precise predictions when the interactions can be treated as a small perturbation. However, in the complementary case of strong coupling, while QFT often gives the correct qualitative explanation of the observed phenomena, it lacks a systematic approach to compute observables from first principles. Relatedly, we do not have yet a general understanding of QFT that covers both weak and strong coupling regimes, and encompasses all the possible observables, ranging from scattering amplitude, to correlation functions of local operators, to those of extended operators. Finding better ways to compute will likely also lead to a better understanding of what QFT really is.

Head of Research Group

Head:

Lorenzo Di Pietro

Info

Research

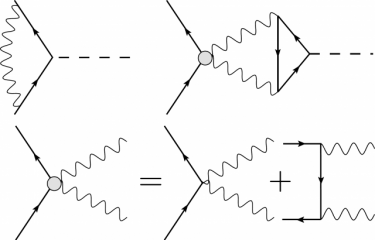
Our research focusses on several interrelated aspects of the QFT framework, such as:

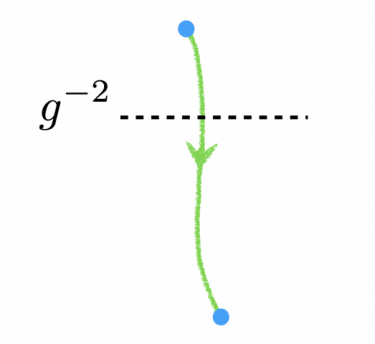
Conformal gauge theories: strongly-coupled gauge theories in various spacetime dimensions d can admit a renormalization group flow connecting them to an interacting conformal field theory (CFT). Gauge theories typically come in families parametrized by the number of colors, the number of matter flavors, and possibly additional parameters such as Chern-Simons couplings. It is not known for what values of these parameters the CFT phase of the gauge theory exists. In d=3 these conformal gauge theories have application to certain second order transitions with persistent order. In d=5 they are the most promising candidate to provide examples of interacting CFTs without supersymmetry, yet unknown. We investigate this problem using resummations of the perturbative expansion, and the epsilon-expansion to approach gauge theories in d=3 and d=5 spacetime dimensions.

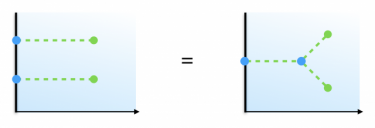
Boundary conditions: studying QFT in spaces with boundaries defines a large set of new observables, the boundary correlation functions for a given boundary condition. Boundary conditions can preserve some symmetry of the bulk theory, such as conformal symmetry, in which case they can be used to describe surface transitions in statistical physics, or supersymmetry. Given a certain QFT, it is an open problem to classify the possible boundary conditions that preserve certain symmetries. We study this problem for simple bulk CFTs, such as free massless fields, and for supersymmetric theories where some observables can be computed exactly.

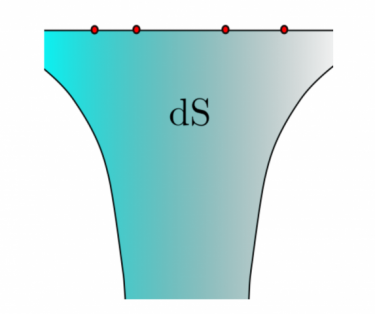
Quantum field theory in curved space: placing a QFT in a curved background can be used to regulate the infrared behavior and have more control on the dynamics. A particularly nice example is that of the Euclidean Anti-De Sitter (EAdS) space, which in many aspects behaves like a finite-size box, but on the other hand retains a large spacetime symmetry and allows to define asymptotic observables akin to scattering amplitudes in flat space, thanks to the presence of a boundary. We study aspects of QFT in EAdS, going beyond the perturbative regime via large N and boundary CFT techniques. We also use QFT in EAdS as a tool to compute late-time correlators in De Sitter (dS) space, which describes a maximally symmetric expanding universe, having in mind applications to inflationary correlators.

People

PhD students: Ankur, Davide Bason

Local collaborators: Roberto Valandro, Marco Serone (SISSA), Fabiana De Cesare (SISSA), Riccardo Ciccone (SISSA)

Publications

Recent publications:

C. Behan, L. Di Pietro, E. Lauria, B. van Rees, “Bootstrapping boundary-localized interactions II: Minimal models at the boundary”, JHEP 03 (2022) 146, arXiv:2111.04747 [hep-th],
L. Di Pietro, M. Mariño, M. Sberveglieri, M. Serone, “Resurgence and 1/N Expansion in Integrable Field Theories”, JHEP 10 (2021) 166, arXiv:2108.02647 [hep-th],
L. Di Pietro, V. Gorbenko, S. Komatsu, “Analyticity and Unitarity for Cosmological Correlators”, JHEP 03 (2022) 023, arXiv:2108.01695 [hep-th],
F. De Cesare, L. Di Pietro, M. Serone, “Five-dimensional CFTs from the ε-expansion”, Phys.Rev. D104 (2021) 105015, arXiv:2107.00342 [hep-th],
L. Di Pietro, E. Lauria, P. Niro, “3d Large N Vector Models at the Boundary”, SciPost Phys. 11 (2021) 050, arXiv:2012.07733 [hep-th],
C. Behan, L. Di Pietro, E. Lauria, B. van Rees “Bootstrapping boundary-localized interactions”, JHEP 12 (2020) 182, arXiv:2009.03336 [hep-th],
S. Chapman, L. Di Pietro, K. Grosvenor, Z. Yan “Renormalization of Galilean Electrodynamics”, JHEP 10 (2020) 195, arXiv:2007.03033 [hep-th],
L. Di Pietro, M. Serone “Looking through the QCD Conformal Window with Perturbation Theory”, HEP 07 (2020) 049, arXiv:2003.01742 [hep-th],
C. Closset, L. Di Pietro, H. Kim “’t Hooft anomalies and the holomorphy of supersymmetric partition functions”, JHEP 1908 (2019) 035, arXiv:1905.05722 [hep-th],
L. Di Pietro, D. Gaiotto, E. Lauria and J. Wu “3d Abelian Gauge Theories at the Boundary”, JHEP 1905 (2019) 091, arXiv:1902.09567 [hep-th],
D. Carmi, L. Di Pietro, and S. Komatsu, “A Study of Quantum Field Theories in AdS at Finite Coupling”, JHEP 1901 (2019) 200, arXiv: 1810.04185 [hep-th].

Last update: 09-11-2024 - 07:45

Hide/Show